题面：

Error Curves

Input file: standard input

Output file: standard output

Time limit: 2 second

Memory limit: 256 megabytes

 

Josephina is a clever girl and addicted to Machine Learning recently. She 

pays much attention to a method called Linear Discriminant Analysis, which

has many interesting properties.

In order to test the algorithm's efficiency, she collects many datasets.

What's more, each data is divided into two parts: training data and test

data. She gets the parameters of the model on training data and test the

model on test data. To her surprise, she finds each dataset's test error curve is just a parabolic curve. A parabolic curve corresponds to a quadratic function. In mathematics, a quadratic function is a polynomial function of the form f(x) = ax^2 + bx + c. The quadratic will degrade to linear function if a = 0.

It's very easy to calculate the minimal error if there is only one test error curve. However, there are several datasets, which means Josephina will obtain many parabolic curves. Josephina wants to get the tuned parameters that make the best performance on all datasets. So she should take all error curves into account, i.e., she has to deal with many quadric functions and make a new error definition to represent the total error. Now, she focuses on the following new function's minimum which related to multiple quadric functions. The new function F(x) is defined as follows: F(x) = max(Si(x)), i = 1...n. The domain of x is [0, 1000]. Si(x) is a quadric function. Josephina wonders the minimum of F(x). Unfortunately, it's too hard for her to solve this problem. As a super programmer, can you help her?

 

 

Input

The input contains multiple test cases. The first line is the number of cases T (T < 100). Each case begins with a number n (n ≤ 10000). Following n lines, each line contains three integers a (0 ≤ a ≤ 100), b (|b| ≤ 5000), c (|c| ≤ 5000), which mean the corresponding coefficients of a quadratic function.

 

Output

For each test case, output the answer in a line. Round to 4 digits after the decimal point.

 

Example

Input

2

1

2 0 0

2

2 0 0

2 -4 2

Output

0.0000

0.5000

 

题目描述：

给出多个二次函数S(x)，第i个函数为Si(x)，求F(x)在定义域[0, 1000]内的最小值。

 

题目分析：

这题需要动手画个图：

当有两个二次函数S1(x),S2(x)时，可能有下面的情况：

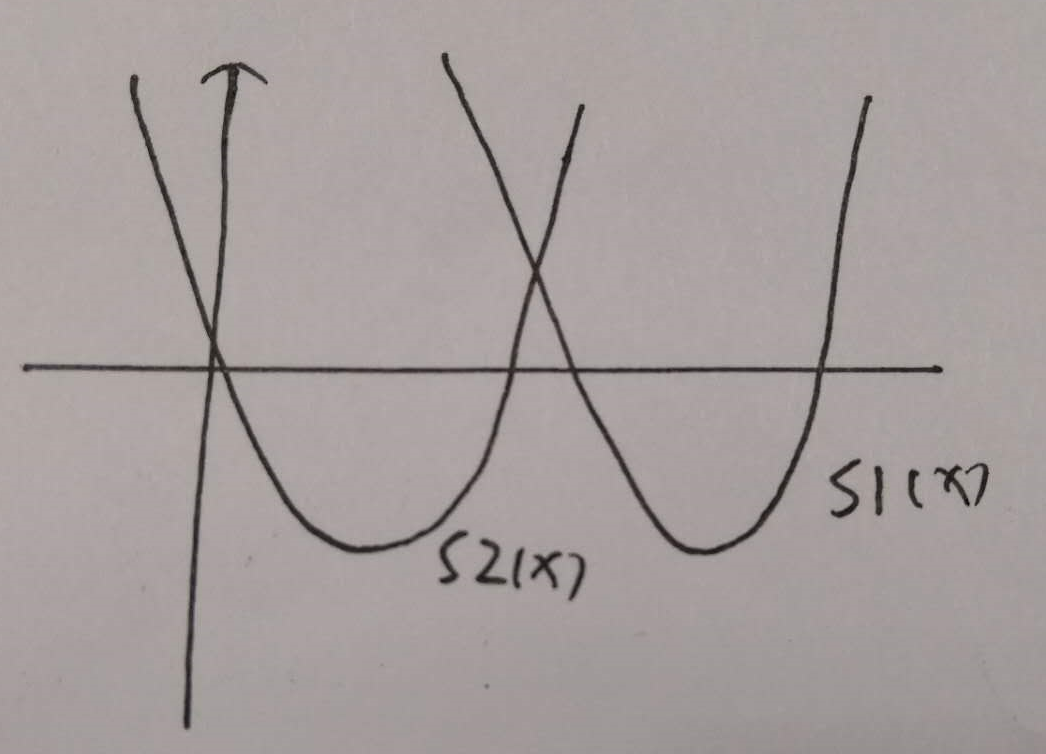
所以F(x)为：

当有三个函数时，F(x)为这样：

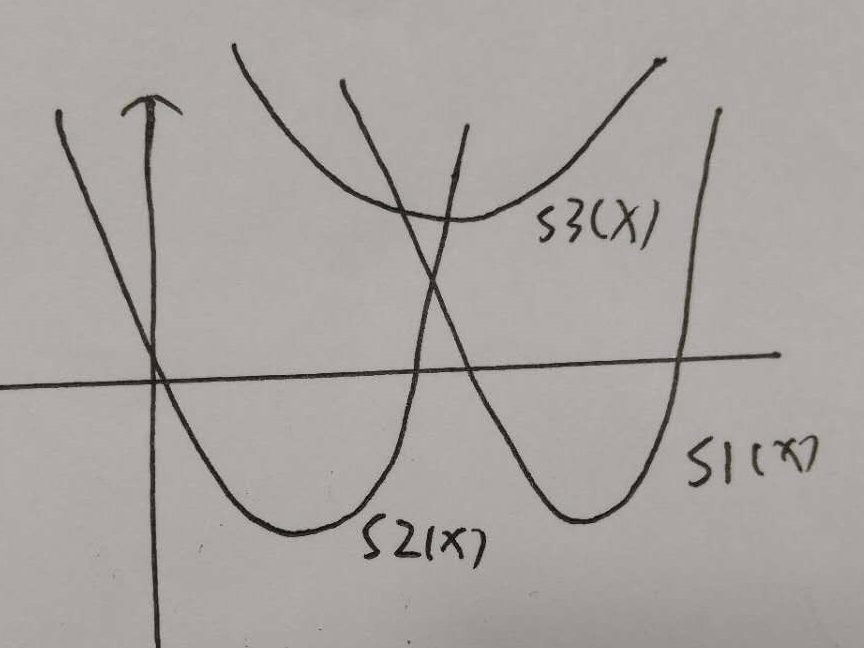
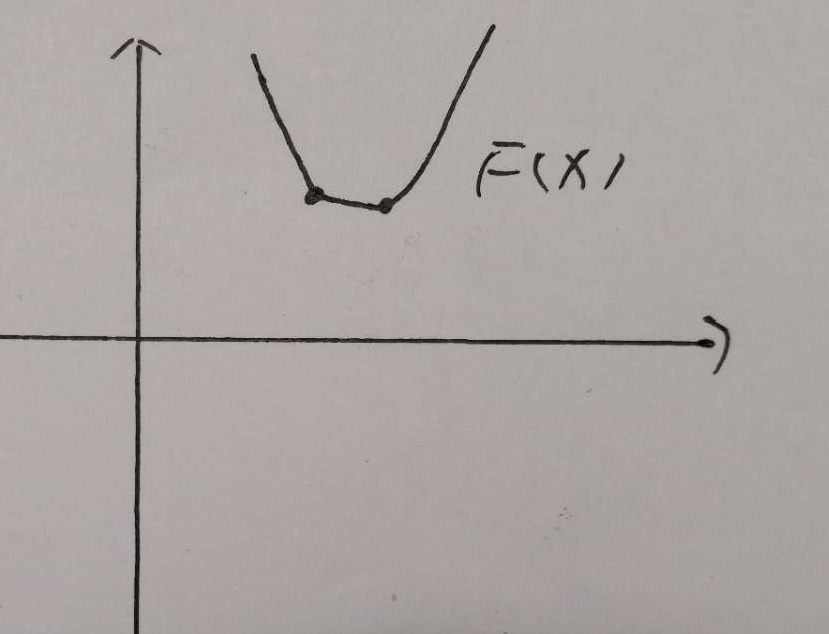
通过观察，我们可以发现：无论有多少个二次函数Si(x)，F(x)一定是向下“凹”的。所以，利用这一点，我们可以套上的模板。

但是，这个F(x)的式子都不知道，怎样计算F(x)？当然是按照F(x)的定义来算啦：直接遍历所有的二次函数，取最大值就行了。

最后要注意：这里的精度至少要设置为1e-9才行，1e-8及以上会WA。

 

AC代码：

1 #include 
      
        2 #include 
       
         3 #include 
        
          4 #include 
         
           5 using namespace std; 6 const int maxn = 10000 + 5; 7 int a[maxn], b[maxn], c[maxn]; 8 int n; 9 10 double F(double x){  //直接按定义算F(x)11     double mmax = -1e9;12     double temp;13     for(int i = 0; i < n; i++){14         temp = a[i]*x*x+b[i]*x+c[i];15         mmax = max(mmax, temp);16     }17     return mmax;18 }19 20 int main(){21     int t;22     cin >> t;23     while(t--){24         //清空数组25         memset(a, 0, sizeof(a));26         memset(b, 0, sizeof(b));27         memset(c, 0, sizeof(c));28         29         cin >> n;30         for(int i = 0; i < n; i++){31             cin >> a[i] >> b[i] >> c[i];32         }33         34         //三分模板35         double L = 0, R = 1000;36         double eps = 1e-10;37         double mid, p;38         while(fabs(R-L) > eps){39             mid = (L+R)/2;40             p = (mid+R)/2;41             if(F(p) > F(mid)) R = p;42             else L = mid;43         }44         45         printf("%.4lf\n", F(L));46     }47     return 0;48 }